How to Write Slope Intercept Form of an Equation
What is slope-intercept form? This article is here to help!
Read below to learn how to write equations in slope-intercept form. We'll also discover how to find slope-intercept form from two points, from a slope and a point, and from a graph. Additionally, we will see how to determine the x-intercepts and y-intercepts.

Changing an equation into a certain form can help us to identify useful information. Just like we can change play-dough or clay from one shape to another, we can change equations to reveal the information we need. Let's learn more about slope-intercept form!
What is slope-intercept form?
The slope-intercept form of an equation is:
y=mx+b
What is the b?
The variable b represents the y-intercept. This is where the line crosses the y-axis.
What is the m?
The variable m represents the slope.
Remember, slope of a linear equation is often described as \frac{\text{rise}}{\text{run}}. For more info on slope, visit our review article on how to find slope.
Return to top
Slope-intercept equation from two points (example)
To determine how to write an equation from two points, we must determine the value of the slope and the y-intercept. First, we will calculate the slope. This will be the value of m in the slope-intercept form equation:
y=mx+b
Let's answer the question, "What is the slope-intercept form of a line going through the points (1,5) and (-4,7)?"
Remember, to calculate the slope we use the equation:
\dfrac{y_2-y_1}{x_2-x_1}
First, we can label the points.
Now, we can substitute the values into the slope equation.
\dfrac{y_2-y_1}{x_2-x_1}
\dfrac{7-5}{-4-1}
\dfrac{2}{-5}
\dfrac{-2}{5}
Therefore, the slope of the line is \frac{-2}{5}. We can substitute \frac{-2}{5} for m.
y=mx+b
y=\dfrac{-2}{5}x+b
Now, we must determine the value of b. To do so, we will use one point and substitute the values of x and y. Let us use the point (1,5). We will substitute 1 for x and 5 for y.
y=\dfrac{-2}{5}x+b
5=\dfrac{-2}{5}1+b
5=\dfrac{-2}{5}+b
5+\dfrac{2}{5}=b
\dfrac{25}{5}+\dfrac{2}{5}=b
\dfrac{27}{5}=b
Therefore, the value of b is \frac{27}{5}, which is the y-intercept. We will now substitute \frac{27}{5} for b.
y=\dfrac{-2}{5}x+b
y=\dfrac{-2}{5}x+\dfrac{27}{5}
For an additional example of writing an equation from two points, watch the video below:
Return to top
Slope-intercept equation given slope and y-intercept (example)
Instead of being given two points, we may need to know how to find slope-intercept form with slope and the y-intercept. In this example, we will use a slope of -4 and a y-intercept of \frac{1}{5}.
Remember, the slope is represented by m and the y-intercept is represented by b. We will simply substitute the given values for m and b. We start with form:
y=mx+b
y=-4x+\dfrac{1}{5}
Return to top
Find slope-intercept equation from graph (example)
Similarly, when we need to know how to write slope-intercept form from a graph, we determine the slope and the y-intercept.
When presented with a graph, we must first determine two points on the grid lines and identify those points.
For our graph, we will use the points (1,1) and (0,-2). Now, we determine the rise and the run. Slope is \frac{\text{rise}}{\text{run}}. For us, the rise is how far up we must travel to get from (0,-2) to get to (1,1). The run is how far to the right we must travel to get from (0,-2) to get to (1,1). Let us determine these values by counting on the graph.
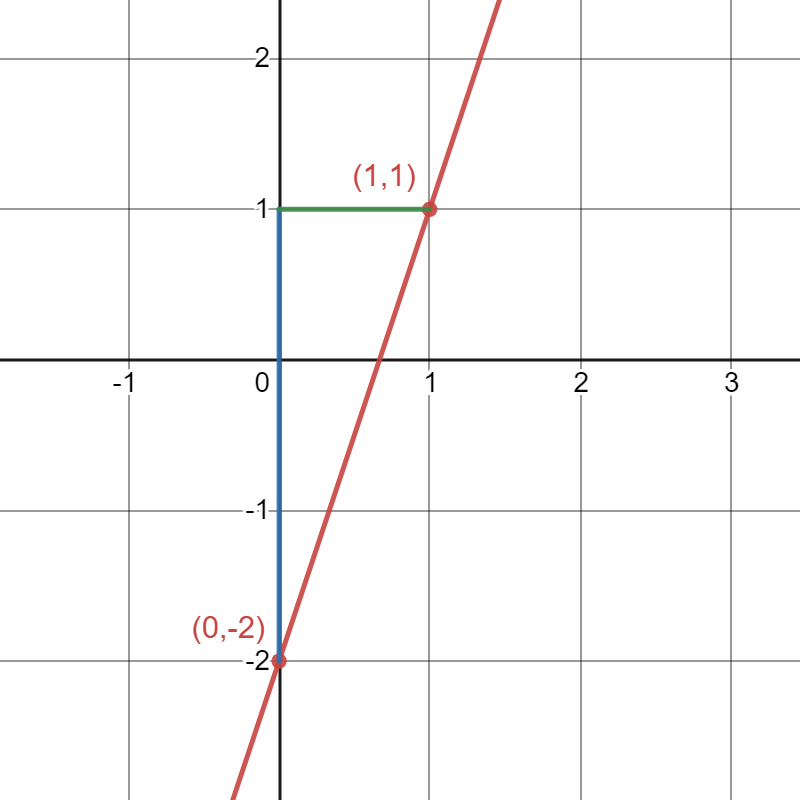
- The rise, the vertical change shown in blue, is 3.
- The run, the horizontal change shown in green, is 1.
- Therefore, the slope is \frac{\text{rise}}{\text{run}} or \frac{3}{1}
This means, the slope of the line is 3.
The y-intercept is where the line crosses the y-axis, the vertical axis. In this case, the line crosses the y-axis at the point (0,-2), so the y-intercept is -2.
Because the slope is 3 and the y-intercept is -2, we will substitute 3 for m and -2 for b to create the slope-intercept form of the equation.
y=mx+b
y=3x+(-2)
y=3x-2
If you're a visual learner, below is a brief video example of writing a slope-intercept equation from a graph:
Return to top
Calculate x and y intercepts from slope-intercept form (example)
Consider the slope-intercept form equation y=6x+9. We can determine the x and y-intercepts. The x-intercept occurs when y equals 0, and the y-intercept occurs when x equals 0. We can determine the x-intercept by setting the value of y equal to 0.
y=6x+9
0=6x+9
-9=6x
\dfrac{-9}{6}=x
\dfrac{-3}{2}=x
Therefore, the x-intercept of the equation is \frac{-3}{2}. This means the graph will cross the x-axis when x equals \frac{-3}{2}.
Because the equation is written in slope-intercept form, we can easily determine the y-intercept. The y-intercept is the value of b in the equation.
y=mx+b
y=6x+9
Because we can see that 9 is the value of b, we know that the y-intercept of the equation is 9.
Return to top
Other forms of linear equations
Linear equations can also be written in point-slope form, determined by one point on the line and the slope of the line. To learn more, read our detailed review article on point-slope form.
y-y_1=m(x-x_1)
A linear equation can also be written in standard form. This form can be very useful to solve systems of equations. To learn more, read our review guide on the standard form of linear equations.
ax+by=c
Return to top
Summary: Slope-Intercept Form
- Remember, slope-intercept form is: y=mx+b .
- We have determined slope-intercept form using a graph, using a point and a slope, and using two points.
- We have also found x and y-intercepts from an equation in slope-intercept form.
Looking for video summary of this content? Checkout this helpful 5-minute video explanation of slope-intercept form.
Click here to explore more helpful Albert Algebra 1 review guides.
Return to top
How to Write Slope Intercept Form of an Equation
Source: https://www.albert.io/blog/slope-intercept-form/
0 Response to "How to Write Slope Intercept Form of an Equation"
Post a Comment